
If Factor B is nested within Factor A, then a level ofįactor B can only occur within one level of Factor A and there can be no In this case,Įach operator is not crossed with each machine but rather only runs one An example of this might be if we want to study the effects ofĭifferent machines and different operators on some output characteristic,īut we can't have the operators change the machines they run.

When fewer than all levels of one factor occur within each level of the otherįactor. Sometimes, constraints prevent us from crossing every level of oneįactor with every level of the other factor. Residual standard error: 0.5995 on 97 degrees of freedom
#Anova degrees of dom calculator code
Just to show you that the F statistic is the same: run the following code (I have generated a bunch of random uniform variables to make a regression, but you can replace it with your own variables and it should be the same) > a|t|) Or maybe that's exactly what you want, to just compare your model against the intercept only model, which is what it's doing but a single significant t test would tell you that the F test would therefore have to be significant anyway. But the point is just look at the t values and you can see which one is significant-within the model anyway. On this case, anyone of a, b, or c could be having an effect.
#Anova degrees of dom calculator full
To compare full vs reduced models you are removing only one variable and seeing if that affects the sums of squares explained by the new model. That is not the same as comparing full vs reduced models. In other words, if R is doing the first thing (comparing to a null with just an intercept), then I think this is contrary to what I've been taught, but if the latter (comparing to multiple nulls, each test being a single degree of freedom comparison), then I don't think there is a problem.įrom your elaboration, it appears you want to know what the F statistic means when you summary(lm(x~a+b+c))?īasically, it tells you the difference between the model x~a+b+c compared to the intercept only model x~1. Is there any point in doing the comparison? Null = lm(x ~ b + c, data=mydata) #compact modelĪnova(null, model) # single degree-of-freedom comparison between augmented model and compact model.īut this latter approach isn't very often taught, particularly when one is using R, though Bodo Winter seems to be an exception. So, if I was interested in the effect of a, then I was taught to compare the augmented model with the compact model as follows: model = lm(x ~ a + b + c, data=mydata) #augmented model I am worried because I was taught to compare models with only a single degree of freedom between them, ie an augmented model that includes the parameter of interest against a compact model that excludes the parameter of interest.
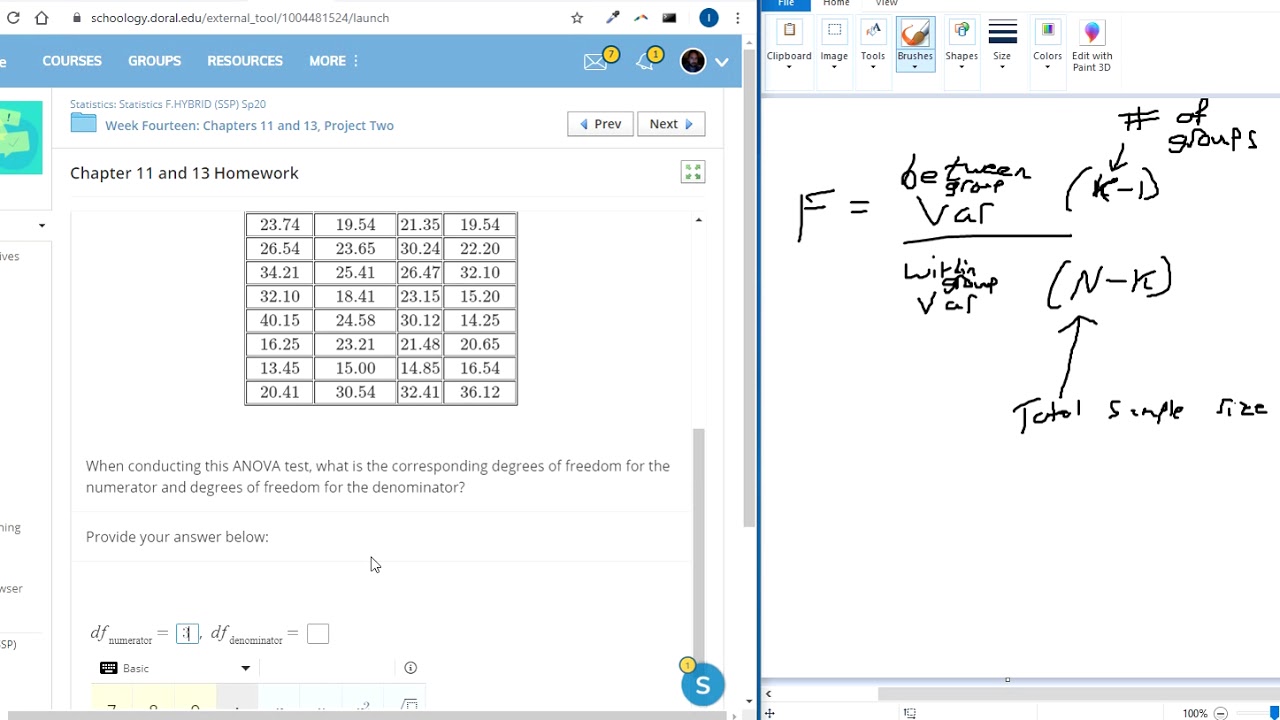
Null3=lm(x ~ a + b, data=mydata) # compact model3 Null2=lm(x ~ a + c, data=mydata) # compact model2 Null1=lm(x ~ b + c, data=mydata) # compact model1 Or is it doing multiple single-degree-of-freedom tests, such as comparing: model = lm(x ~ a + b + c, data=mydata) # augmented model Is R only doing an omnibus test and comparing my augmented model to a null model with only an intercept: null=lm(x ~ 1, data=mydata) # compact model Assume we have a model in R: model = lm(x ~ a + b + c, data=mydata) # augmented model I would like to know whether R produces single degrees of freedom tests for a formula.


 0 kommentar(er)
0 kommentar(er)
